So sánh P với căn bậc hai của P

Hướng dẫn cách rút gọn biểu thức chứa căn cho học sinh lớp 9. Đây là dạng toán chắc chắn có trong các đề thi tuyển sinh vào lớp 10 môn Toán.

Để giải được bài tập rút gọn và tính giá trị của biểu thức chứa căn bậc hai chúng ta cần ôn lại lý thuyết căn thức bậc hai. Tức là:

Biến đổi biểu thức chứa căn bậc hai

Vận dụng các quy tắc dưới đây:

1. Đưa thừa số ra ngoài dấu căn

Với hai biểu thứcmà, ta có; tức là:

Nếuvàthì

2. Đưa thừa số vào trong dấu căn

Vớivàthì

3. Khử mẫu của biểu thức lấy căn

Với hai biểu thứcmàvàta có:

$\displaystyle \sqrt{\frac{A}{B}}=\frac{\sqrt{A.B}}{\left| B \right|}$

4. Trục căn thức ở mẫu

Với hai biểu thức A, B màB >0 ta có:

$\displaystyle \frac{A}{\sqrt{B}}=\frac{A\sqrt{B}}{B}$

Với các biểu thứcmàvàta có:

$\displaystyle \frac{C}{\sqrt{A}\pm B}=\frac{C(\sqrt{A}\pm B)}{A-B_{{}}^{2}}$

Với các biểu thứcmàvàta có:

$\displaystyle \frac{C}{\sqrt{A\pm \sqrt{B}}}=\frac{C(\sqrt{A}\pm B)}{A-B}$

Bài tập: Thực hiện phép tính chứa căn bậc hai dưới đây:

1);

2) $\displaystyle \frac{{10+2\sqrt{{10}}}}{{\sqrt{5}+\sqrt{2}}}+\frac{8}{{1-\sqrt{5}}}$ ;

3);

4) $\displaystyle \frac{{2\sqrt{8}-\sqrt{{12}}}}{{\sqrt{{18}}-\sqrt{{48}}}}-\frac{{\sqrt{5}+\sqrt{{27}}}}{{\sqrt{{30}}+\sqrt{{162}}}}$ ;

5) $\displaystyle \sqrt{{\frac{{2-\sqrt{3}}}{{2+\sqrt{3}}}}}+\sqrt{{\frac{{2+\sqrt{3}}}{{2-\sqrt{3}}}}}$ ;

6) $\displaystyle 2\sqrt{{\frac{{16}}{3}}}-3\sqrt{{\frac{1}{{27}}}}-6\sqrt{{\frac{4}{{75}}}}$ ;

7) $\displaystyle 2\sqrt{{27}}-6\sqrt{{\frac{4}{3}}}+\frac{3}{5}\sqrt{{75}}$ ;

8) $\displaystyle \frac{{\sqrt{{3-\sqrt{5}}}.\left( {3+\sqrt{5}} \right)}}{{\sqrt{{10}}+\sqrt{2}}}$

9);

10) $\displaystyle \sqrt{{2-\sqrt{3}}}\left( {\sqrt{5}+\sqrt{2}} \right)$ ;

11);

12) $\displaystyle \sqrt{{4+\sqrt{{10+2\sqrt{5}}}}}+\sqrt{{4-\sqrt{{10+2\sqrt{5}}}}}$ ;

13) $\displaystyle \left( {5+2\sqrt{6}} \right)\left( {49-20\sqrt{6}} \right)\sqrt{{5-2\sqrt{6}}}$ ;

14) $\displaystyle \frac{1}{{\sqrt{2}+\sqrt{{2+\sqrt{3}}}}}+\frac{1}{{\sqrt{2}-\sqrt{{2-\sqrt{3}}}}}$ ;

15) $\displaystyle \frac{{6+4\sqrt{2}}}{{\sqrt{2}+\sqrt{{6+4\sqrt{2}}}}}+\frac{{6-4\sqrt{2}}}{{\sqrt{2}-\sqrt{{6-4\sqrt{2}}}}}$ ;

16) $\displaystyle \frac{{{{{\left( {\sqrt{5}+2} \right)}}^{2}}-8\sqrt{5}}}{{2\sqrt{5}-4}}$ ;

17);

18) $\displaystyle \frac{4}{{\sqrt{3}+1}}+\frac{1}{{\sqrt{3}-2}}+\frac{6}{{\sqrt{3}-3}}$ ;

19) $\displaystyle {{\left( {\sqrt{2}+1} \right)}^{3}}-{{\left( {\sqrt{2}-1} \right)}^{3}}$

20) $\displaystyle \frac{{\sqrt{3}}}{{1-\sqrt{{\sqrt{3}+1}}}}+\frac{{\sqrt{3}}}{{1+\sqrt{{\sqrt{3}+1}}}}$ .

Phương pháp rút gọn:
Phân tích đa thức tử và mẫu thành nhân tử;
Tìm ĐKXĐ (Nếu bài toán chưa cho ĐKXĐ)
Rút gọn từng phân thức (nếu được)
Thực hiện các phép biến đổi đồng nhất như:
+ Quy đồng (đối với phép cộng trừ) ; nhân ,chia.
+ Bỏ ngoặc: bằng cách nhân đơn ; đa thức hoặc dùng hằng đẳng thức
+ Thu gọn: cộng, trừ các hạng tử đồng dạng.
+ Phân tích thành nhân tử rút gọn

* Chú ý: Trong mỗi bài toán rút gọn thường có các câu thuộc các loại toán: Tính giá trị biểu thức; giải Phương trình; bất phương trình; tìm giá trị của biến để biểu thức có giá trị nguyên; tìm giá trị nhỏ nhất ,lớn nhấtDo vậy ta phải áp dụng các Phương pháp giải tương ứng, thích hợp cho từng loại bài.

Ví dụ: Cho biểu thức: $\displaystyle P=\left( {\frac{1}{{a-\sqrt{a}}}+\frac{1}{{\sqrt{a}-1}}} \right):\frac{{\sqrt{a}+1}}{{a-2\sqrt{a}+1}}$

a/ Rút gọn.

b/ Tìm giá trị củađể biểu thứccó giá trị nguyên.

Giải:

a/ Rút gọn:

Phân tích: $\displaystyle P=\left[ {\frac{1}{{\sqrt{a}(\sqrt{a}-1)}}+\frac{1}{{\sqrt{a}-1}}} \right]:\frac{{\sqrt{a}+1}}{{{{{(\sqrt{a}-1)}}^{2}}}}$

ĐKXĐ: $\displaystyle \left\{ \begin{array}{l}a0;\\\sqrt{a}-1\ne 0\Leftrightarrow a\ne 1\end{array} \right.$

Quy đồng: $\displaystyle P=\frac{{1+\sqrt{a}}}{{\sqrt{a}(\sqrt{a}-1)}}.\frac{{{{{(\sqrt{a}-1)}}^{2}}}}{{\sqrt{a}+1}}$

Rút gọn: $\displaystyle P=\frac{{\sqrt{a}-1}}{{\sqrt{a}}}.$

b/ Tìm giá trị củađểcó giá trị nguyên:

Chia tử cho mẫu ta được: $\displaystyle P=1-\frac{1}{{\sqrt{a}}}$ .

Lý luận:nguyên $\displaystyle \Leftrightarrow \frac{1}{{\sqrt{a}}}$ nguyênlà ước của 1 là. $\Rightarrow \sqrt{a}=\left\{ \begin{array}{l}-1(ktm)\\1\Leftrightarrow a=1\end{array} \right.$

Vậy vớithì biểu thứccó giá trị nguyên.

Bài tập rút gọn, tính giá trị biểu thức chứa căn lớp 9:

Bài 1: Cho biểu thức $\displaystyle A =\left( \frac{\sqrt{x}}{2}-\frac{1}{2\sqrt{x}} \right)\left( \frac{x-\sqrt{x}}{\sqrt{x}+1}-\frac{x+\sqrt{x}}{\sqrt{x}-1} \right)$

a. Rút gọn biểu thức;

b. Tìm giá trị củađể.

Bài 2: Cho biểu thức $\displaystyle B =\left( {\frac{{\sqrt{x}}}{{x-4}}+\frac{2}{{2-\sqrt{x}}}+\frac{1}{{\sqrt{x}+2}}} \right):\left( {\sqrt{x}-2+\frac{{10-x}}{{\sqrt{x}+2}}} \right)$

a) Rút gọn biểu thức;

b) Tìm giá trị củađể.

Bài 3: Cho biểu thức: $\displaystyle C =\frac{1}{{\sqrt{x}-1}}-\frac{3}{{x\sqrt{x}+1}}+\frac{1}{{x-\sqrt{x}+1}}$

a) Rút gọn biểu thức;

b) Tìm giá trị củađể.

Bài 4: Rút gọn biểu thức:

$\displaystyle D =\frac{{x+2+\sqrt{{{{x}^{2}}-4}}}}{{x+2-\sqrt{{{{x}^{2}}-4}}}}+\frac{{x+2-\sqrt{{{{x}^{2}}-4}}}}{{x+2+\sqrt{{{{x}^{2}}-4}}}}$

Bài5: Cho các biểu thức:

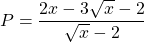$\displaystyle P =\frac{{2x-3\sqrt{x}-2}}{{\sqrt{x}-2}}$ và

a) Rút gọn biểu thứcvà;

b) Tìm giá trị củađể.

Bài 6: Cho biểu thức: 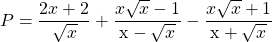$\displaystyle P =\frac{{2x+2}}{{\sqrt{x}}}+\frac{{x\sqrt{x}-1}}{{\text{x}-\sqrt{x}}}-\frac{{x\sqrt{x}+1}}{{\text{x}+\sqrt{x}}}$

a) Rút gọn biểu thức

b) So sánhvới.

c) Với mọi giá trị củalàmcó nghĩa, chứng minh biểu thứcchỉ nhận đúng một giá trị nguyên.

Bài 7: Cho biểu thức:

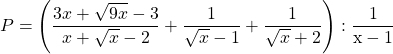
$\displaystyle P =\left( {\frac{{3x+\sqrt{{9x}}-3}}{{x+\sqrt{x}-2}}+\frac{1}{{\sqrt{x}-1}}+\frac{1}{{\sqrt{x}+2}}} \right):\frac{1}{{\text{x}-1}}$

a) Tìm điều kiện đểcó nghĩa, rút gọn biểu thức;

b) Tìm các số tự nhiên x đểlà số tự nhiên;

c) Tính giá trị củavới.

Bài 8: Cho biểu thức : $\displaystyle P =\left( {\frac{{\sqrt{x}+2}}{{\text{x}-5\sqrt{x}+6}}-\frac{{\sqrt{x}+3}}{{\text{2}-\sqrt{x}}}-\frac{{\sqrt{x}+2}}{{\sqrt{x}-3}}} \right):\left( {2-\frac{{\sqrt{x}}}{{\sqrt{x}+1}}} \right)$

a) Rút gọn biểu thức;

b/Tìm x để 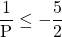$\displaystyle \frac{1}{\text{P}}\le -\frac{5}{2}$

Bài 9: Cho biểu thức : 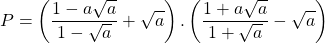$\displaystyle P = \left( {\frac{{1-a\sqrt{a}}}{{1-\sqrt{a}}}+\sqrt{a}} \right).\left( {\frac{{1+a\sqrt{a}}}{{1+\sqrt{a}}}-\sqrt{a}} \right)$

a) Rút gọn

b) Tìm a để

Bài 10: Cho biểu thức: 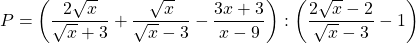$\displaystyle P = \left( {\frac{{2\sqrt{x}}}{{\sqrt{x}+3}}+\frac{{\sqrt{x}}}{{\sqrt{x}-3}}-\frac{{3x+3}}{{x-9}}} \right):\left( {\frac{{2\sqrt{x}-2}}{{\sqrt{x}-3}}-1} \right)$

a) Rút gọn

b) Tìm x để 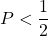$\displaystyle P \frac{1}{2}$

c) Tìm giá trị nhỏ nhất của

Bài 11: Cho biểu thức : 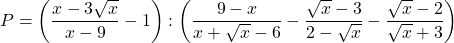$\displaystyle P = \left( {\frac{{x-3\sqrt{x}}}{{x-9}}-1} \right):\left( {\frac{{9-x}}{{x+\sqrt{x}-6}}-\frac{{\sqrt{x}-3}}{{2-\sqrt{x}}}-\frac{{\sqrt{x}-2}}{{\sqrt{x}+3}}} \right)$

a) Rút gọn

b) Tìm giá trị củađể

Bài 12: Cho biểu thức : 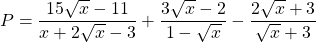$\displaystyle P = \frac{{15\sqrt{x}-11}}{{x+2\sqrt{x}-3}}+\frac{{3\sqrt{x}-2}}{{1-\sqrt{x}}}-\frac{{2\sqrt{x}+3}}{{\sqrt{x}+3}}$

a) Rút gọn

b) Tìm các giá trị củađể 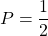$\displaystyle P= \frac{1}{2}$

c) Chứng minh 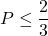$\displaystyle P \le \frac{2}{3}$

Bài 13: Cho biểu thức: 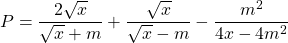$\displaystyle P = \frac{{2\sqrt{x}}}{{\sqrt{x}+m}}+\frac{{\sqrt{x}}}{{\sqrt{x}-m}}-\frac{{{{m}^{2}}}}{{4x-4{{m}^{2}}}}$ với m > 0

a) Rút gọn

b) Tínhtheođể.

c) Xác định các giá trị củađểtìm được ở câu b thoả mãn điều kiện

Bài 14: Cho biểu thức : 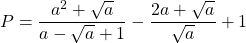$\displaystyle P = \frac{{{{a}^{2}}+\sqrt{a}}}{{a-\sqrt{a}+1}}-\frac{{2a+\sqrt{a}}}{{\sqrt{a}}}+1$

a) Rút gọn

b) Tìmđể

c) Tìm giá trị nhỏ nhất của?

Bài 15: Cho biểu thức:

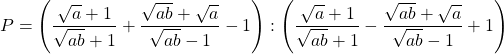
$\displaystyle P=\left( {\frac{{\sqrt{a}+1}}{{\sqrt{{ab}}+1}}+\frac{{\sqrt{{ab}}+\sqrt{a}}}{{\sqrt{{ab}}-1}}-1} \right):\left( {\frac{{\sqrt{a}+1}}{{\sqrt{{ab}}+1}}-\frac{{\sqrt{{ab}}+\sqrt{a}}}{{\sqrt{{ab}}-1}}+1} \right)$

a) Rút gọn

b) Tính giá trị củanếuvà 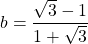$\displaystyle b = \frac{{\sqrt{3}-1}}{{1+\sqrt{3}}}$

c) Tìm giá trị nhỏ nhất củanếu

Bài 16: Cho biểu thức:

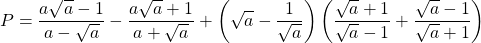
$\displaystyle P=\frac{{a\sqrt{a}-1}}{{a-\sqrt{a}}}-\frac{{a\sqrt{a}+1}}{{a+\sqrt{a}}}+\left( {\sqrt{a}-\frac{1}{{\sqrt{a}}}} \right)\left( {\frac{{\sqrt{a}+1}}{{\sqrt{a}-1}}+\frac{{\sqrt{a}-1}}{{\sqrt{a}+1}}} \right)$

a) Rút gọn

b) Với giá trị nào củathì

c) Với giá trị nào củathì

Bài 17: Cho biểu thức: 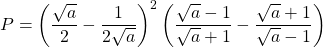$\displaystyle P = {{\left( {\frac{{\sqrt{a}}}{2}-\frac{1}{{2\sqrt{a}}}} \right)}^{2}}\left( {\frac{{\sqrt{a}-1}}{{\sqrt{a}+1}}-\frac{{\sqrt{a}+1}}{{\sqrt{a}-1}}} \right)$

a) Rút gọn

b) Tìm các giá trị củađể

c) Tìm các giá trị củađể

Bài 18: Cho biểu thức: 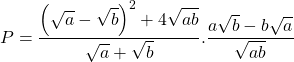$\displaystyle P = \frac{{{{{\left( {\sqrt{a}-\sqrt{b}} \right)}}^{2}}+4\sqrt{{ab}}}}{{\sqrt{a}+\sqrt{b}}}.\frac{{a\sqrt{b}-b\sqrt{a}}}{{\sqrt{{ab}}}}$

a) Tìm điều kiện đểcó nghĩa.

b) Rút gọn

c) Tính giá trị củakhi a =và b =

Bài 19: Cho biểu thức: 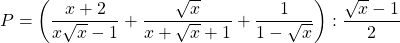$\displaystyle P = \left( {\frac{{x+2}}{{x\sqrt{x}-1}}+\frac{{\sqrt{x}}}{{x+\sqrt{x}+1}}+\frac{1}{{1-\sqrt{x}}}} \right):\frac{{\sqrt{x}-1}}{2}$

a) Rút gọn

b) Chứng minh rằngvới

Bài 20: Cho biểu thức : 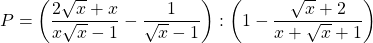$\displaystyle P = \left( {\frac{{2\sqrt{x}+x}}{{x\sqrt{x}-1}}-\frac{1}{{\sqrt{x}-1}}} \right):\left( {1-\frac{{\sqrt{x}+2}}{{x+\sqrt{x}+1}}} \right)$

a) Rút gọn

b) Tínhkhi x =

Bài 21: Cho biểu thức: 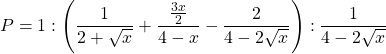$\displaystyle P = 1:\left( {\frac{1}{{2+\sqrt{x}}}+\frac{{\frac{{3x}}{2}}}{{4-x}}-\frac{2}{{4-2\sqrt{x}}}} \right):\frac{1}{{4-2\sqrt{x}}}$

a) Rút gọn

b) Tìm giá trị củađể

Bài 22: Cho biểu thức : 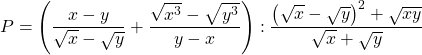$\displaystyle P = \left( {\frac{{x-y}}{{\sqrt{x}-\sqrt{y}}}+\frac{{\sqrt{{{{x}^{3}}}}-\sqrt{{{{y}^{3}}}}}}{{y-x}}} \right):\frac{{{{{\left( {\sqrt{x}-\sqrt{y}} \right)}}^{2}}+\sqrt{{xy}}}}{{\sqrt{x}+\sqrt{y}}}$

a) Rút gọn

b) Chứng minh P

Bài 23: Cho biểu thức :

$\displaystyle P = \left( {\frac{1}{{\sqrt{a}+\sqrt{b}}}+\frac{{3\sqrt{{ab}}}}{{a\sqrt{a}+b\sqrt{b}}}} \right).\left[ {\left( {\frac{1}{{\sqrt{a}-\sqrt{b}}}-\frac{{3\sqrt{{ab}}}}{{a\sqrt{a}-b\sqrt{b}}}} \right):\frac{{a-b}}{{a+\sqrt{{ab}}+b}}} \right]$

a) Rút gọn

b) Tính P khivà

Bài 24: Cho biểu thức: 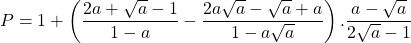$\displaystyle P = 1+\left( {\frac{{2a+\sqrt{a}-1}}{{1-a}}-\frac{{2a\sqrt{a}-\sqrt{a}+a}}{{1-a\sqrt{a}}}} \right).\frac{{a-\sqrt{a}}}{{2\sqrt{a}-1}}$

a) Rút gọn

b) Cho 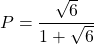$\displaystyle P = \frac{{\sqrt{6}}}{{1+\sqrt{6}}}$ tìm giá trị của a

c) Chứng minh rằng 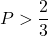$\displaystyle P \frac{2}{3}$

Bài 25: Cho biểu thức: 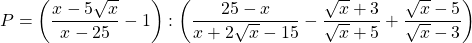$\displaystyle P = \left( {\frac{{x-5\sqrt{x}}}{{x-25}}-1} \right):\left( {\frac{{25-x}}{{x+2\sqrt{x}-15}}-\frac{{\sqrt{x}+3}}{{\sqrt{x}+5}}+\frac{{\sqrt{x}-5}}{{\sqrt{x}-3}}} \right)$

a) Rút gọn

b) Với giá trị nào củathì

Bài 26: Cho biểu thức:

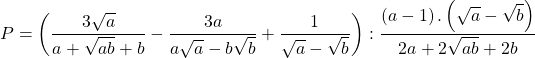
$\displaystyle P = \left( {\frac{{3\sqrt{a}}}{{a+\sqrt{{ab}}+b}}-\frac{{3a}}{{a\sqrt{a}-b\sqrt{b}}}+\frac{1}{{\sqrt{a}-\sqrt{b}}}} \right):\frac{{\left( {a-1} \right).\left( {\sqrt{a}-\sqrt{b}} \right)}}{{2a+2\sqrt{{ab}}+2b}}$

a) Rút gọn

b) Tìm những giá trị nguyên củađểcó giá trị nguyên

Bài 27: Cho biểu thức: 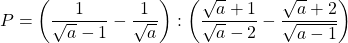$\displaystyle P = \left( {\frac{1}{{\sqrt{a}-1}}-\frac{1}{{\sqrt{a}}}} \right):\left( {\frac{{\sqrt{a}+1}}{{\sqrt{a}-2}}-\frac{{\sqrt{a}+2}}{{\sqrt{{a-1}}}}} \right)$

a) Rút gọn

b) Tìm giá trị củađể 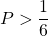$\displaystyle P \frac{1}{6}$

Bài 28: Cho biểu thức:

$\displaystyle P=\left[ {\left( {\frac{1}{{\sqrt{x}}}+\frac{1}{{\sqrt{y}}}} \right).\frac{2}{{\sqrt{x}+\sqrt{y}}}+\frac{1}{x}+\frac{1}{y}} \right]:\frac{{\sqrt{{{{x}^{3}}}}+y\sqrt{x}+x\sqrt{y}+\sqrt{{{{y}^{3}}}}}}{{\sqrt{{{{x}^{3}}y}}+\sqrt{{x{{y}^{3}}}}}}$

a) Rút gọn

b) Cho. Xác địnhđể P có giá trị nhỏ nhất

Bài 29: Cho biểu thức: 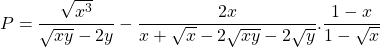$\displaystyle P = \frac{{\sqrt{{{{x}^{3}}}}}}{{\sqrt{{xy}}-2y}}-\frac{{2x}}{{x+\sqrt{x}-2\sqrt{{xy}}-2\sqrt{y}}}.\frac{{1-x}}{{1-\sqrt{x}}}$

a) Rút gọn

b) Tìm tất cả các số nguyên dương x để y=625 và P<0,2

Bài 30: Cho biểu thức: 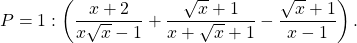$\displaystyle P = 1:\left( {\frac{{x+2}}{{x\sqrt{x}-1}}+\frac{{\sqrt{x}+1}}{{x+\sqrt{x}+1}}-\frac{{\sqrt{x}+1}}{{x-1}}} \right).$

a) Rút gọn

b) So sánhvới

Cùng chuyên đề:Đại số 9 - Tags: biểu thức, rút gọn biểu thức, toán 9

So sánh P với căn bậc hai của P

Hướng dẫn cách rút gọn biểu thức chứa căn cho học sinh lớp 9. Đây là dạng toán chắc chắn có trong các đề thi tuyển sinh vào lớp 10 môn Toán.

Biến đổi biểu thức chứa căn bậc hai

Bài tập rút gọn, tính giá trị biểu thức chứa căn lớp 9:

Video liên quan

Responsive Ad

Billboard Ad

Biểu mẫu liên hệ